Fraunhofer Institute for Digital Medicine MEVIS
Clinical Challenge
An accurate and comprehensive diagnosis is required for optimal treatment to restore joint stability and patient-specific joint kinematics. However, the functionality of the joint can only be properly quantified by analyzing the dynamics, which is not (yet) part of today’s clinical practice. It is therefore necessary and important to also include kinematic quantification in order to find the best therapy.
Solution & Feature
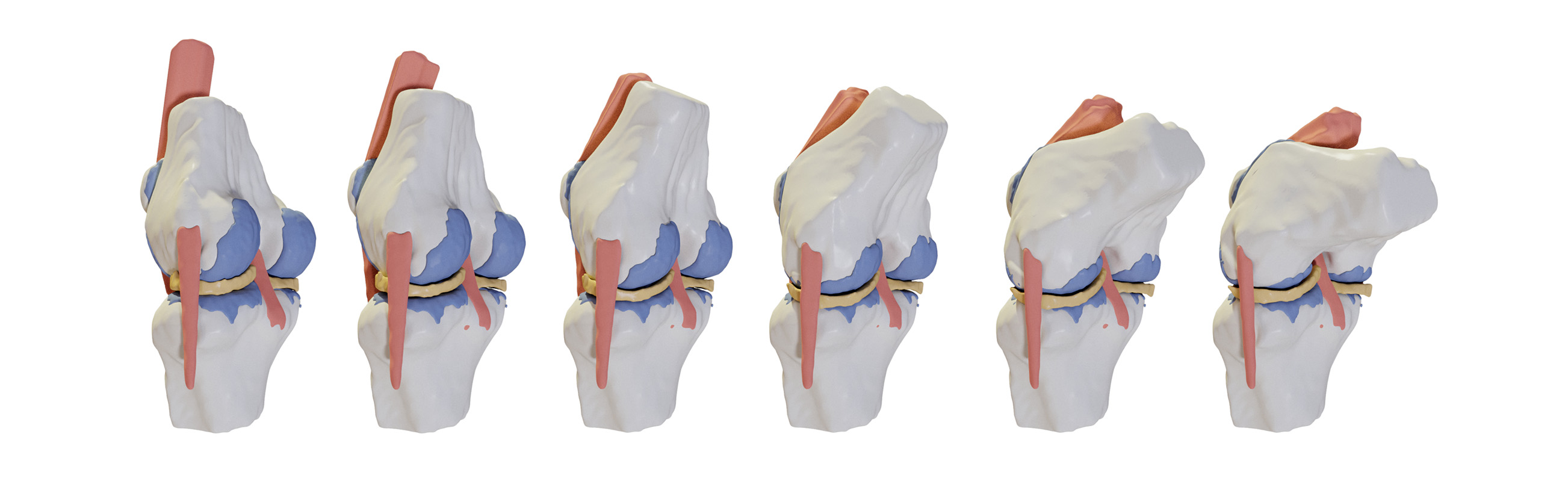
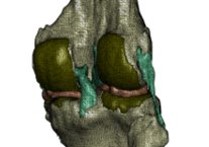
From magnetic resonance images of the injured joint, a dynamic and patient-specific biomechanical model can be derived. The evaluation of individual joint kinematics - such as pressure distribution on the cartilage or stresses in ligaments - can be incorporated into the clinical diagnostic and therapeutic process. The simulation of ligament reconstructions, joint implants or osteotomy enables virtual planning of surgical treatment in advance with the aim of achieving the best approximation to the physiological and pre-traumatic joint kinematics.
Fraunhofer MEVIS develops solutions for intervention planning by using:
- Semi-automatic segmentation of involved anatomies
- Position-based dynamics for interactive kinematic analysis
- Finite Element Simulation for detailed joint analysis
- Coupling of simulations with motion tracking data
- Quantification of clinical assessments
- Efficient GPU Implementations
- Statistical shape models
Highlights
We focus on incorporating patient-individual information into a solution driven by the clinical needs and workflows.
- Project PLANK: knee kinematic quantification and restoration using patient-specific biomechanic models
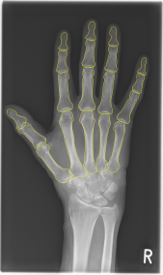
- Project FingerKIt
- BRIDGE: software technology using deformation simulation (Finite Element Model)
- Best paper award